News Archive
Our former colleague Florian Faucher started his ERC Starting Grant ``Passive imaging in terrestrial and solar seismology''i on January 1st 2024.
»Now MSc Research Interships at the University of Pau and Pays L-Adour are open.
We are happy to announce that Leopold Veselka, PhD student in the Sub-project Quantitative Coupled Physics Imaging of the «SFB Tomography Across the Scales», has successfully defended his PhD thesis with the title “Reconstruction of optical parameters in quantitative optical coherence tomography” on 9th of January, 2024.
Leopold’s doctoral study was supervised by Peter Elbau, the principal investigator of the Sub-project Quantitative Coupled Physics Imaging affiliated with the Faculaty of Mathematics, University of Vienna. Congratulations from the SFB colleagues!
During a special session of the Chemnitz Symposium on Inverse Problems 2023, Otmar Scherzer gave a talk commemorating the 70th birthday of Bernd Hofmann, founder of the Symposium in 2002.
» press release (german)
Thursday, October 5, 15:30
SP 416-2
RICAM, Altenberger Str. 69, 4040 Linz
Optical imaging uses visible or near-infrared light to interrogate internal properties of biological tissues based on endogenous (for example oxygenated and deoxygenated haemoglobin) or exogenous (contrast agents) contrast. Several optical imaging modalities have been developed, and these techniques are of great interest due to the special contrast that rises from the physiological nature of light absorbing molecules. One example of tomographic imaging techniques based on light is diffuse optical tomography. In diffuse optical tomography, distributions of optical parameters inside an imaged target are estimated from light transport measurements made on its boundary. This is a highly ill-posed inverse problem, and the technique can be used to provide images with a unique contrast. However, it suffers from a low resolution due to the diffuse behaviour of light in biological tissues. Utilising so-called coupled physics imaging can overcome the limitations of diffuse imaging modalities. Perhaps the most developed of these coupled techniques is photoacoustic imaging, that combines the contrast of light with the resolution of ultrasound utilising the photoacoustic effect. In this talk, I will discuss tomography using light and ultrasound with the focus on modelling and inverse problems. Principles of diffuse optical tomography and photoacoustic tomography together with the applications are reviewed. Furthermore, problem of quantitative tomography is discussed.
Within the FWF-funded Hertha-Firnberg-Program «Photoacoustic Tomography: Analysis and Numerics», we offer a Doctorate Research Position (20 Months with a possible extension of up to 4 years) at Johann Radon Institute for Computational and Applied Mathematics of the Austrian Academy of Sciences (Linz)
Within the FWF-funded International Project «New Inverse Problems in Superresolution Microscopy», we offer a Post Doc Research Position, 3 years at the Faculty of Mathematics, University of Viennai
We also off a Doctorate Research Position (2 years with a possible extension of up to 4 years) at the Faculty of Mathematics, University of Vienna
Otmar Scherzer will be a speaker at the CIMPA Research Summer School on "Mathematical Modelling and Numerical Simulation in Medicine", which will be held at the Universidad de La Habana, Cuba, on 4-7 June 2023. There, he will give lectures virtually on topics in inverse problems: an introductory lecture on tomography and a more advanced lecture on numerical inversion. » further details
Otmar Scherzer gave a talk on «Newton methods for solving linear inverse problems with neural network coders» at the colloquium in Linz celebrating the 20th anniversary of the Johann Radon Instute (RICAM) and the 70th birthday of its founder, Prof. Heinz W. Engl.
Prof. Heinz W. Engl also gave an interview in which he was asked about how research has developed since his inception of the institute and what his own personal research highlights are.
David Omogbhe receives SIAM travel award for the 2023 SIAM Conference on Computational
Science and Engineering [1] (CSE23) to take place in Amsterdam, The
Netherlands from February 26 - March 3, 2023. Congratulations!
On November 16, 9am CET, Otmar Scherzer
gave a talk
«Inverse Problems for Acoustic Attenuating Media»
on recent results obtained in the
CD-Laboratory for Mathematical Modelling and Simulation of Next-Generation Medical UltraSound Devices
and the
SFB Tomography Across the Scales.
Currently, the autumn school as part of the Special Semester «Tomography Across the Scales» takes places at the RICAM in Linz. Peter Elbau, also one of our project leaders of the SFB «Tomography Across the Scales», was invited to give an introductory lecture on the mathematics behind optical coherence tomography. The audience, including several members of CSC, warmly welcomed the intuitive and very well presented lecture on this highly complex topic.
The CD laboratory «MaMSi» and Otmar Scherzer are featured in an article in the newspaper «DerStandard» (German).
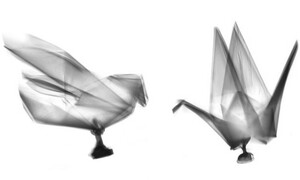
We are happy to announce that the educational paper Computed Origami Tomography by Axel Kittenberger, Leonidas Mindrinos and Otmar Scherzer got published in SIAM Review.
The authors demonstrate the principles of computer tomography using harmless light instead of x-rays. With the provided instructions, building your own DIY-tomograph at home is very much possible. See for 3D reconstructions of an origami crane as exemplary result.
We kindly invite you to the talk "Concentration inequalities for cross-validatin in scattered data approximation" by Felix Bartel, Apr. 14th, 14:00pm Vienna Time.
Join Zoom Meeting: https://oeaw-ac-at.zoom.us/j/92440653189?pwd=cm1KVCtSaHViTXNjYkxuYVpNVU9qUT09
Meeting-ID: 924 4065 3189
Password: fY9hzA
We present a new factorization method for recovering a conductivity inclusion in two dimensions from multi-static measurements. A conductivity inclusion induces a perturbation in the background potential, and the perturbation admits a multipole expansion whose coefficients are the so-called generalized polarization tensors (GPTs). We derive a factorization formula for the matrix composed of the GPTs in terms of the material parameters and the coefficients of the exterior conformal mapping associated with the inclusion. Using this formula, we induce accurate representations for the coefficients of the conformal mapping in terms of the GPTs. Our approach provides a non-iterative method for recovering the shape of a Lipschitz inclusion with arbitrary finite conductivity.
We are offering a Post Doc Research/Coordination Position within on Special Research Programmes (SFB F68) Tomography Across the Scales at the Faculty of Mathematics, University of Vienna, funded by the Austrian Science Fund (FWF) (https://www.fwf.ac.at/de/). The position is full time, 40 hours per week, for an initial period of two year with a possible extension till 28. February 2026.
We kindly invite you to the talk "Adaptive spectral decomposition for inverse medium problems" by Marcus J. Grote, March 30, 2:00pm Vienna Time.
This is part of the Joint Fudan - RICAM Seminar on Inverse Problems
The meeting is a hybrid meeting, come either in person to the meeting room 9 at Oskar-Morgenstern-Platz 1, 9th floor or
join the zoom meeting: https://zoom.us/join Meeting-ID: 694 9293 2905 Password: SCMS
Abstract: Inverse medium problems involve the reconstruction of a spatially varying unknown medium from available observations of a scattered wave field. Typically, they are formulated as PDE-constrained optimization problems and solved by an inexact Newton-like iteration. Clearly, standard grid-based representations are very general but often too expensive due to the resulting high-dimensional search space. Adaptive spectral inversion (ASI) instead expands the unknown medium in a basis of eigenfunctions of a judicious elliptic operator, which depends itself on the current iterate. Thus, instead of a grid-based discrete representation combined with standard Tikhonov regularization, the unknown medium is projected to a small finite-dimensional subspace, which is iteratively adapted using dynamic thresholding. Rigorous error estimates of the adaptive spectral decomposition (ASD) are proved for an arbitrary piecewise constant medium.
Within the FWF-funded International Project New Inverse Problems in Superresolution Microscopy, we offer a Doctorate Research Position (2 years with a possible extension of up to 4 years) at the Faculty of Mathematics, University of Vienna The position is available from July 1st, 2022. The duties concern research in inverse problems related to superresolution microscopy, regularization methods and mathematical modeling. Publications in high quality journals, networking with partners in applied sciences is desired. The salary is as suggested by the FWF according to a doctorate position. The working load for the project is 30 hours per week.
(→ detailed information)We kindly invite you to the talk "Control problem of a water waves system in a tank" by Pei, Su, Apr. 6th, 3:00pm Vienna Time.
Contact to get an invite link.
We first study the stability of a class of skew-adjoint control systems.
With the assumptions on the spectrum of the evolution operators involved
in the control system, we obtain an explicit non-uniform decay rate of the
energy, provided that the initial data is smooth.
Then we consider a small-amplitude water waves system in a rectangular
domain, where the control acts on one lateral boundary, by imposing the
velocity of the water. The equation is a fully linear and fully dispersive
version of the Zakharov-Craig-Sulem formulation. Based on the Dirichlet to
Neumann and Neumann to Neumann maps, we establish the well-posedness
of the whole system, which is addressed by formulating the equations as an
abstract linear control system. Afterwards, there exists a feedback functional,
such that the corresponding control system is strongly stable. We obtain the
decay rate of the energy by using the above general results.
Finally, we consider the asymptotic behaviour of the above system in
the shallow water regime, i.e. the horizontal scale of the domain is much
larger than the typical water depth. We prove that the solution of the water
waves system converges to the solution of the one dimensional wave equation
with Neumann boundary control, when taking the shallowness limit. Our
approach is based on a detailed analysis of the Fourier series and the di-
mensionless version of the evolution operators mentioned above, as well as a
scattering semigroup and the Trotter-Kato approximation theorem.
This is partially collaborated with M. Tucsnak (Bordeaux) and G. Weiss
(Tel Aviv).
We kindly invite you to the talk "Matrix factorization for the multi-static data and its application to shape recovery of a planar inclusion." by 최두성 Choi, Doosung, Apr. 6th, 1:00pm Vienna Time.
Contact to get an invite link.
We present a new factorization method for recovering a conductivity inclusion in two dimensions from multi-static measurements. A conductivity inclusion induces a perturbation in the background potential, and the perturbation admits a multipole expansion whose coefficients are the so-called generalized polarization tensors (GPTs). We derive a factorization formula for the matrix composed of the GPTs in terms of the material parameters and the coefficients of the exterior conformal mapping associated with the inclusion. Using this formula, we induce accurate representations for the coefficients of the conformal mapping in terms of the GPTs. Our approach provides a non-iterative method for recovering the shape of a Lipschitz inclusion with arbitrary finite conductivity.
We kindly invite you to the talk «Revisiting linear PDEs via the unified transform» by Konstantinos Kalimeris. Monday, Feb. 14, 3:00pm Vienna Time.
Join either online – contact to get an invite link – or in person at SR7, Oskar-Morgenstern-Platz 1, Vienna
In almost every textbook for Partial Differential Equations(PDEs), solutions of linear PDEs for specific initial and boundary value problems (IBVPs) are presented. Many of these problems are considered classical, since they provide a paradigm of how the several methods for solving linear PDEs are applied. In this talk we revisit some of these classical problems using the Unified Transform (UT) method, also known as the Fokas method. Our goal is twofold: (a) show some major limitations of the classical transform methods (b) give the outline of a new unified methodology which overcomes these limitations and provides effective pathways for the relevant analytical and numerical solutions. This talk is also appropriate for undergraduate students with basic knowledge in Complex Analysis and PDEs.
The Vienna International School of Earth and Space Sciences (VISESS) has a vacancy for a fully funded PhD position within the Faculty of Earth Sciences, Geography and Astronomy at the University of Vienna.
This PhD position is part of our interdisciplinary SFB project «Tomography Across the Scales» with the title «Interdisciplinary Advances in Extragalactic Archaeology». Please find more information here.
We kindly invite you to the talk "Individual Function Approximation and Cross-validation " by Felix Bartel. Wednesday, Feb. 9, 3:00pm Vienna Time.
Join either online – contact to get an invite link – or in SR7, Oskar-Morgenstern-Platz 1, Vienna
In this talk we present joint work with Daniel Potts and Ralf Hielscher on scattered data approximation for individual functions (Monte Carlo setting). We show error bounds for the least squares method in the $L_2$-norm which hold with high probability. These bounds are also applicable for noisy samples. The error behavior poses the question of model selection. Here, we utilize leave-one-out cross-validaiton. We show, that the cross-validation score can be computed in a fast manner in this context. Further, we give error guarantees which validate cross-validation in model selection.
The special semester is postponed to 2022, but a smaller numbers of workshops will still take place. (→ more information).
We kindly invite you to the talk "On some inverse source problems governed by fractional diffusion equations" by Mohamed Ben Salah. Monday, Oct. 4th, 9:00am Vienna Time.
Contact to get an invite link.
This topic is concerned with inverse source problems related to fractional operators. Three problems have been addressed in this research study.
The first one is related to the fractional Laplacian operator. The aim is to reconstruct an unknown source term from internal noisy measured data. The leading term of the mathematical model equation is governed by the fractional spectral Laplacian. The inverse problem is formulated as a regularized optimiza- tion one minimizing a least square type functional. The existence, uniqueness and stability of the unknown source term have been established. In the numer- ical part, we develop a numerical reconstruction approach for identifying the unknown source term and solving the inverse problem.
The second inverse problem is governed by a time-fractional diffusion equa- tion. It consists in identifying an unknown source term support from boundary measurements of the potential field. In this study, we have proposed a fast and accurate approach combining the robustness of the Kohn-Vogelius formulation and the rapidity of the topological gradient method. In the theoretical part of this work, We have derived a topological asymptotic expansion, with respect to a small geometric perturbation of the source term, valid for a large class of source functions. In the numerical part, we have developed a reconstruction algorithm for solving the considered geometric inverse source problem and iden- tifying the location, size and shape of the unknown sub-domains.
The third inverse source problem is related to a space-time fractional diffu- sion equation. Our aim is to identify an unknown source term from partially observed data. The employed model involves the Caputo fractional derivative in time and the non-local fractional Laplacian operator in space. The well- posedness of the forward problem is discussed. The considered ill-posed inverse source problem is formulated as a minimization one. The existence, uniqueness and stability of the solution of the minimization problem are examined. An iter- ative process is developed for identifying the unknown source term. A numerical implementation of the proposed approach is performed. The convergence of the discretized fractional derivatives is analyzed. The efficiency and accuracy of the proposed identification algorithm are confirmed by some numerical experiments.
We kindly invite you to the talk "A realistic electrode model in electrical impedance tomography" by Noemi Naujoks. Monday, Sep. 27th, 10:00am Vienna Time.
Contact to get an invite link.
Electrical impedance tomography (EIT) is a non-invasive imaging technique based on the reconstruction of electrical conductivities in the human body. For this purpose, various current patterns are injected into the body via electrodes and the resulting voltages are measured. From a mathematical point of view, the reconstruction of conductivity using these measured data provides an ill-posed, non-linear inverse problem. The task is to include the use of electrodes into the mathematical model. The gap model describes the simplest type of electrode modeling by assuming that the current, injected through a specific electrode, has a uniform strength on the entire area of the electrode. However, in reality, the distribution of a current flow along an electrode is unknown. Furthermore, electrodes are usually built from a highly conductive material, which we consider a perfect conductor. Hence the potential along each electrode is assumed to be constant. The shunt model, which forms a more realistic approach considering these properties, is investigated in this work. To perform the reconstruction of conductivity using this model, an inexakt Newton-type method is applied. The aim is to implement the theoretical findings and investigate whether the realistic electrode modeling can achieve a positive effect in reconstruction. A comparison of the numerical results of the two models indicates that the shunt model is more accurate at reconstructing images than the gap model.
The Tenth International Conference "Inverse Problems: Modelling and Simulation" (IPMS 2022) will be held during May 22-28, 2022 at the Congress Center of the Paradise Bay Hotel, Mellieha, Malta. The IPMS conference series is one of the main scientific meetings of the field which has been organized every two years since 2002, under the auspices of The Eurasian Association on Inverse Problems (EAIP). The Conference IPMS 2022 is the tenth (Jubilee) in the series. The objective of this meeting is to be multidisciplinary and international, bringing together scientists working on various topics of inverse problems in diverse areas. An important goal of the IPMS conferences is to encourage the participation of young researchers by offering them the opportunity to deliver invited talks and by partially supporting them. We look forward to welcoming you to Malta.
(→ more information)We kindly invite you to the talk "On Some Inverse Problems: Boundary Data Completion and Buried Flaws Detection" by Naima Ben Meghnia. Friday, Aug. 6th, 10:00am Vienna Time.
Online at: https://meet.csc.univie.ac.at/b/axe-ick-ugy-pcx
This presentation focuses, on the one hand, on the reconstruction of missing boundary data using the fictitious domain decomposition technique for the heat equation via a new robust algorithm that exploits the dynamic in time and we investigate the convergence analysis. On the other hand, on the identification of cavities in the two-dimensional linear elasticity system from the knowledge of partially overdetermined boundary data using the topological sensitivity analysis. we obtain the explicit formula of the topological derivative taking the energy-gap and L2-gap as cost functions, then we compare both functionals numerically. Several numerical experiments are illustrated to highlight the robustness and the accuracy of these two approaches.
We are inviting to the Workshop "Tomographic Reconstructions and their Startling Applications" at the Erwin Schrödinger Institute for Mathematics and Physics (ESI) from March 15 until March 26. Due to the current restrictions, the conference will be held as an online event.
The workshop is organized within the interdisciplinary research program "Tomography Across the Scales" and is centered around the mathematical and experimental problems related to the applications studied in this project, involving adaptive optics, optical coherence tomography, photoacoustics, and superresolution imaging.
We kindly invite you to the talk "Regularising linear inverse problems under unknown non-Gaussian noise" by Tim Jahn. Wednesday, Jan. 27th, 13:00 Vienna Time.
Online at: https://meet.csc.univie.ac.at/b/axe-7hr-2ad
We deal with the solution of linear ill-posed equations in Hilbert spaces. Usually, one only has a corrupted measurement of the right hand side at hand and the Bakushinskii veto tells us, that we are not able to solve the equation if we do not know the noise level. But in applications such ad hoc knowledge may often be unrealistic. However, the error of a measurement may often be estimated through averaging of repeated measurements. We combine this with classical Filter-based regularisation methods for a purely data-driven regularisation scheme. We obtain convergence to the true solution, with the only assumption that the measurements are unbiased, independent and identically distributed according to an elseways arbitrary unknown distribution (with finite variance or of white noise type). Moreover, we analyse the discrepancy principle as an adaptive stopping rule for a stochastic gradient descent, as a non-Filter based computationally efficient regularisation method.
We kindly invite you to the talk "Sensitivity analysis for identification of voids under Navier's boundary conditions in linear elasticity" by Bochra Mejri. Wednesday, Jan. 27th, 15:00 Vienna Time.
Online at: https://meet.csc.univie.ac.at/b/axe-phh-y2a
This talk is concerned with a geometric inverse problem related to the two-dimensional linear elasticity system. Thereby, voids under Navier’s boundary conditions are reconstructed from the knowledge of partially over-determined boundary data. The proposed approach is based on the so-called energy-like error functional combined with the topological sensitivity method. The topological derivative of the energy-like misfit functional is computed through the topological-shape sensitivity method. Firstly, the shape derivative of the corresponding misfit function is presented. Then, an explicit solution of the fundamental boundary-value problem in the infinite plane with a circular hole is calculated by the Muskhelishvili formulae. Finally, the asymptotic expansion of the topological gradient is derived explicitly with respect to the nucleation of a void. Numerical tests are performed in order to point out the efficiency of the developed approach.
We kindly invite you to the talk "Mathematics of Biomimetics for Active Electro-sensing" by Andrea Scapin. Wednesday, Jan. 13th, 15:00 Vienna Time.
Online at: https://meet.csc.univie.ac.at/b/axe-zjv-dya
The biological behavior of weakly electric fish has been studied by scholars for years. These fish orient themselves at night in complete darkness by using electrosensory information, which makes these animals an ideal subject for developing bio-inspired imaging techniques. Such interest has motivated a huge number of studies addressing the active electro-sensing problem from many different perspectives since Lissmann and Machin’s work. One of the most noteworthy potential bio-inspired applications is in underwater robotics. Building autonomous robots with electro-sensing technology may supply unexplored navigation, imaging and classification capabilities, especially when the sight is unreliable due, for example, to the turbidity of the surrounding waters or the poor lighting conditions.
In this talk we present a premier and innovative (real-time) multi-scale method for target classification in electro-sensing. The intent is that of mimicking the behavior of the weakly electric fish, which is able to retrieve much more information about the target by approaching it. The method is based on a family of transform-invariant shape descriptors computed from generalized polarization tensors (GPTs) reconstructed at multiple scales. The evidence provided by the different descriptors at each scale is fused using Dempster-Shafer Theory.
Congratulations to Christopher Hofmann – working within within the SCIP project – for successfully defended his dissertation "Contributions to regularization theory and practice of certain nonlinear inverse problems"!
We're currently offering a PhD position within the framework of Vienna School of Mathematics on the topic of Optimal Control of Waves in Dead Water. (→ detailed information)
We are proud to report that since the online publication of the Handbook of Mathematical Methods in Imaging (Second Edition) in 2015 there have been a total of 175366 chapter downloads!
Otmar Scherzer presented at the Research Newsletter (July/August 2020) of the University of Vienna how mathematics can be used to improve cancer diagnosis. This work is part of the SFB research project Tomography Across the Scales. The considered methods have applications from astrophysics to molecular biology.
The main idea (also called inverse problem) is to use tomographic measurements of a biological tissue in order to recover its properties (distinguish between healthy and diseased parts) without damaging it. The created algorithms are tested with simulated and experimental data and the results are promising.
Every two years the Eurasian Association on Inverse Problems (EAIP) awards scientists under the age of fourty for particular merits in inverse problems analysis and its applications.
We are proud to announce Peter Elbau has been selected with Joonas Ilmavirta to receive this award in 2020!
Last week (02.03 – 06.03) took place in Kefermarkt the first winter school of the Austrian Study Foundation (Österreichische Studienstiftung). That was the fourth seminar organized by the Austrian Academy of Sciences (Österreichische Akademie der Wissenschaften) with the title “Applied mathematics: as useful as exciting”.
Selected pupils from schools around Austria had the opportunity to learn and develop themselves applications of mathematics in everyday life. The four topics where: Financial mathematics, mathematical methods in Tomography, graph theory and mathematical modeling of sound.
Axel Kittenberger and Leonidas Mindrinos, from our group, presented the fundamentals of the Radon transform, its properties and application to tomography. The students had the opportunity to make 3D origami objects, image them with a system imitating an optical tomographic setup and then obtain the reconstructed pictures.
–> click here for an online raytracer of a volumetric reconstruction
A figure from the research paper "Preconditioning Inverse Problems for Hyperbolic Equations with Applications to Photoacoustic Tomography" by Alexander Beigl, Otmar Scherzer, Jarle Sogn (JKU, Linz) and Walter Zulehner (JKU, Linz) got chosen for the front cover of the International Journal "Inverse Problems". The picture appearing at the Volume 36, Number 1 of the journal presents initial source reconstructions from Photoacoustic Tomography data.
Otmar Scherzer presented in "Kronenzeitung" within the weekly column "Krone der Wissenschaft" the ideas and the scopes of the SFB project "Tomography Across the Scales". The article (in german) addresses the general principles of tomography (computed and photoacoustic). The article can be acquired on PressReader.
Otmar Scherzer was a member of the organizing committee of the “Reconstruction Methods for Inverse Problems” workshop in Banff, Canada, June 23-28, 2019. This workshop brought together researchers working on inverse problems coming from medical imaging, non destructive testing, computer vision and geophysics. The PI of sub-project “Quantitative Coupled Physics Imaging”, Peter Elbau and the post-doctoral researcher of the sub-project “Tomography with Uncertainties”, Ekaterina Sherina presented their recent work related to our SFB project “Tomography Across the Scales”.
“Die Presse” printed an an interview with Otmar Scherzer on combining PAT and OCT to detect tumors. (“innovationorigins” published an english version of similar content).
This research is being done within the SFB Tomography Across the Scales.Otmar Scherzer gave an interview (german) about making cancer visible early using a combination of PAT and OCT.
We are offering a Doctorate Research Position within the FWF-funded International Project Novel Error Measure and Source Conditions of Regularization Methods.
RICAM, Linz, is offering a PhD Student position in the field of Inverse Problems / Tomography.
The CSC team participiated at the Vienna Night Run. Despite a sudden cold snap everyone finished!
at Banff, Alberta from Sunday, June 23 to Friday June 28, 2019
Organizers
- Elena Beretta (NYU Abu Dhabi & Politecnico di Milano)
- Uri Ascher (University of British Columbia)
- Otmar Scherzer (University of Vienna)
- Luminita Vese (University of California, Los Angeles)
Description
Inverse problems require to determine the cause from a set of indirect observations. Such problems appear in medical imaging, non destructive testing of materials, computerized tomography, source reconstructions in acoustics, computer vision and geophysics, to mention but a few. The 21st century is the golden age of computer imaging: Measurement devices have become enormously powerful and huge amounts of data are recorded at every eye glimpse. Moreover, computer technology has developed to such a high degree of efficiency that the evaluation of such an enormous amount of data has become possible *if* adequate mathematical and computational tools are used.Recently, the community has been exposed to fundamentally new mathematical models (such as learning), which stimulated exciting theoretical developments and new computational algorithms for solving complicated large scale inverse problems. This workshop will survey modern and identify new mathematical and computational developments for tackling such problems.
more detailsThe Eurasian Association on Inverse Problems (EAIP) is a non-governmental organization working to ensure a coordination between inverse problems research groups and scientific schools in Eurasian countries, by providing international conferences, meetings and summer schools on inverse problems, both in theory and applications.
The association has established an "EAIP Award" to recognize outstanding scientific contributions to the field of inverse problems and continuous efforts to foster cooperation between researchers of Eurasian countries.
The SFB “Tomography Across the Scales” with Otmar Scherzer (SFB speaker and subproject leader) and Computational Science Center member Peter Elbau (subproject leader) is presented in the university magazine uni:view (german).
The Radon Institute for Computational and Applied Mathematics in Linz, the Doctoral Program “Computational Mathematics” and the SFB “Tomography across the Scales” are organizing the international workshop Inverse Problems in the ALPS II and Inaugural Meeting of the SFB “Tomography across the Scales” at the Obergurgl University Center.
The SFB Inaugural Meeting starts on March, 18th after dinner and ends on March, 20th, 2018. The workshop “Inverse Problems in the Alps II” (successor of the workshop “Inverse Problems in the Alps” held in March 2016) starts on March, 21st and ends on March, 23rd 2018 after lunch.
more information
The "Standard" published a video interview "Radon-Transformation: Was ein Origami-Vogel mit Mathematik zu tun hat" of Otmar Scherzer explaining the working principle of tomography on a vivid experiment which produces from the recorded shadows of a rotating, transparent origami figure in front of an overhead projector a three-dimensional computer model.